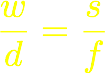Estimating Focal Length

Image Source: Digital Camera World

Without talking to the photographer, we don’t know the length of his/her camera’s focal length or even the type of camera used, but we can come up with a pretty close approximation for f based on estimates of the geometry of the original scene and the size of the image sensor.

We first need an equation to describe the focal length so we know what distances we’ll even need to solve for. Something that will help us is the field of view angle. The horizontal angle encloses the largest object that can fit in the width of the image sensor, and the vertical angle encloses that which fits in its height.

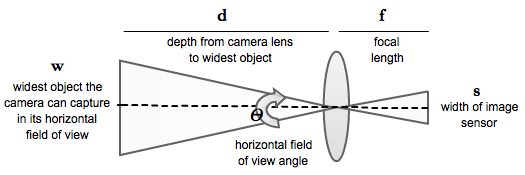
In the above diagram, we see how the dimensions of the largest object captured by the camera are related to the dimensions of the image sensor. We have the image sensor one focal length away from the lens so that the "object distance" is at infinity.

The field of view angle in the horizontal direction can be derived through straight geometry as a function of the object width and depth from the camera on one side of the lens and as a function of the width of the sensor and focal length on the other.

            

We’re then able to equate the ratios and solve for the focal length:

By examining the photograph, we see that the diagonal of the tennis court plus the surrounding stands stretches across the whole width of the image. The depth from that horizontal to the approximate location of the camera will be half the diagonal. But now comes times for the question, how do we estimate that diagonal distance??

While we may not know the dimensions of a tennis court off the top of our heads, we can surely estimate the dimensions of a person. The aim for these types of estimation problems is to keep the calculations simple so as to be able to “do them on the back of a napkin,” so we’ll keep the numbers was to work with and say about one meter per seat. This is definitely an over estimate, but it accounts for the extra space at the ends of the stands.

There seem to be groups of about ten seats, three on the short side and four-five on the long side. Using Pythagorean theorem, we can estimate that the center diagonal is 60 meters and therefore the depth is 30 meters. Finally, we can say that the width of the image sensor in the camera is probably about 2 cm.

From these estimations, we were able to calculate that the focal length was 10mm, which makes sense because lenses with short focal lengths will give the wide-angle perspective that we see in the photograph. And according to the original source, this was in fact the focal length of the lens used!

Further Reading

Optical Insights into Renaissance Art, Falco and Hockney 2000

Physicist Charles M. Falco and artist David Hockney proposed (and tested) a theory that Renaissance painters used optical elements to aid them in their work. Based on the real life dimensions of the various subjects and the size of the canvas, they were able to identify the focal lengths and diameters of the mirrors or lenses that were allegedly used by these artists.